Microgravity Droplet Combustion
General Background for Spherically Symmetric Isolated Droplet Combustion
General Background for Spherically Symmetric Isolated Droplet Combustion
Spherically Symmetric Theory
Since the early 1950's, it has been recognized that the symmetrical burning of an isolated droplet represents
an ideal situation in which to study the complex coupling of chemical reactions and two-phase flow with phase
change. Prior to computers, the simplified geometry of the combustion environment, along with certain
simplifying assumptions concerning physical and chemical processes, permitted mathematical simplifications
of the problem, and led to simple descriptions of the combustion process. Initially, these studies provided
a fundamental foundation upon which to develop more applied, empirical descriptions of spray combustion.
The combustion of a single, isolated liquid droplet in an infinite oxidizing medium is shown schematically
in Figure 1.
In this geometrical configuration, fuel vaporizes at the droplet surface and diffuses outward while oxidizer
diffuses inward from the ambient environment. The fuel and oxidizer react stoichiometrically, resulting in a
zone of intense reaction (i.e. a non-premixed flame). Heat is transported via conduction and radiation
outward from the flame to infinity and inward back to the droplet surface. The heat deposited at the droplet
surface is balanced by the evaporation process at the vapor/liquid interface.
The "classical" d2-law for droplet combustion was first formulated in the 1950s by
assuming that gas-phase chemical reaction is infinitely fast with respect to gas-phase transport, thus
confining chemical reaction to an infinitesimally thin sheet. The assumptions used in this derivation are
shown in Table 1.
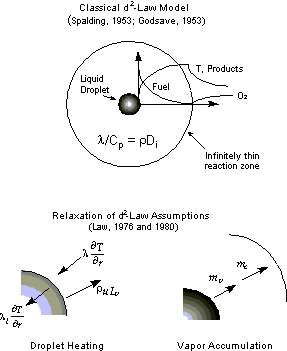
Figure 1 (a) Schematic diagram of classical d2-law droplet combustion model. (b) Relaxations in the d2-law assumptions.
Table1 Assumptions incorporated into the d2-law of droplet combustion.
| Spherical symmetry. | |
| Isolated droplet in infinite medium. | |
| Isobaric process. | |
| Chemical reaction infinitely fast with respect to diffusion. | |
| Constant gas phase transport properties and heat capacity. | |
| Gas phase quasi-steadiness. | |
| Constant, uniform droplet temperature (No droplet heating). | |
| Neglects Soret effect, Dufour effect and radiation. | |
Unity Lewis number for all gaseous species  |
|
| Negligible buoyancy. | |
| Negligible radiation. |
In this geometrical configuration, fuel vaporizes at the droplet surface and diffuses
outward while oxidizer diffuses inward from the ambient environment. The fuel and oxidizer react
stoichiometrically, resulting in a zone of intense reaction (i.e. a non-premixed flame). Heat is transported via
conduction and radiation outward from the flame to infinity and inward back to the droplet surface. The heat
deposited at the droplet surface is balanced by the evaporation process at the vapor/liquid interface. The
"classical" d2-law for droplet combustion was first formulated in the 1950s by assuming
that gas-phase chemical reaction is infinitely fast with respect to gas-phase transport, thus confining chemical
reaction to an infinitesimally thin sheet.
The d2-law theory predicts that the droplet burning rate, flame stand-off position, and flame
temperature remain constant throughout the droplet burning lifetime, and are described by the following
equations:
|
(1) |
|
(2) |
|
(3) |
where, ds is the droplet diameter, 
the thermal
conductivity of the gas, Cp,g the specific heat of the gas, 
the liquid density,
and B the Spalding transfer number, df the flame diameter, Yo, is the ambient oxygen mass
fraction, the stoichiometric oxidizer to fuel mass ratio, Tf the flame temperature, T is the ambient
temperature, Ts the droplet surface temperature, QC the heat of combustion of the liquid
fuel, and H the effective latent heat of vaporization. The Spalding transfer number, B, is a non-dimensional
thermodynamic parameter measuring the ratio of drive toward vaporization through the heat of combustion (along
with the sensible enthalpy difference between the ambient environment and the droplet surface), divided by the
resistance to vaporization through the heat of vaporization:
|
(4) |
For the combustion of most liquid fuels burning in air, the Spalding transfer
number is typically between 1 and 10.
Equations 1 through 3 reproduce experimental observations to varying degrees of success. For single
component droplets, the droplet burning rate is indeed nearly constant in many cases over most of the droplet
lifetime. Also, the qualitative predictions are quite correct as experiments show that the burning rate
increases with increasing 
and decreases with increasing .
Quantitative agreement between experiment and equation 1 can also be achieved provided that appropriate
selections (generally not those determined by the actual physical values) of transport properties are made.
The flame temperature predicted by equation 3 is, essentially, the adiabatic flame temperature of the given
fuel-oxidizer system assuming no dissociation or finite flame thickness. Quantitative agreement, in this case,
can be obtained by assuming a suitably enhanced specific heat to account for deficiencies. The flame stand-off
ratio, which under some experimental conditions can approach a constant value, is vastly over-predicted under
all circumstances by equation 2. That the quantitative agreement is much worse for the flame position than
for the burning rate is easily explained. The assumptions incorporated into the d2-law analysis
yield a flame position which is virtually independent of thermal/transport parameters (generally,
Cp,g(T -Ts) << 
). Thus, there are no parameters to choose
to obtain quantitative agreement. In summary, while the d2-law has historically been shown to be
useful in a qualitative sense, it cannot simultaneously predict the burning rate, flame position and
flame temperature.
Over the years, experimental results have indicated that even some of the qualitative predictions of the
d2-law are in error. For example, during an initial period after ignition, the droplet size actually
changes very little (i.e. the burning rate is initially much lower than would be predicted by the
d2-law). Secondly, the flame standoff ratio is not constant, but rather varies with time. For
environments with low oxygen concentration, the flame standoff continuously increases while, for environments
with higher oxygen concentration, the flame standoff initially increases and then approaches a constant value.
Finally, in many instances, extinction of the envelope diffusion flame has been observed to occur at
finite droplet diameters. Extinction of the diffusion flame results in a rapid decrease in the burning
rate.
By accounting for transient heating of the liquid droplet (Fig. 1b), it is possible to explain the
experimentally observed initial period during which the droplet burning rate is low, even with infinitely fast
chemistry. An energy balance at the droplet surface shows that heat conducted to the surface from the gas phase
balances with heat lost by conduction into the liquid interior and heat lost from the surface due to vaporization
phase change. Initially, when the droplet temperature is low, much of the heat applied to the surface is
conducted inward, resulting in a lower rate of vaporization. Once the droplet heats up toward the liquid
boiling point, little heat is conducted into the liquid interior and the vaporization rate reaches its
quasi-steady value.
Accounting for the accumulation of fuel vapor between the liquid droplet and the flame reproduces
the observed variation in flame position with time (Fig. 1c). In the d2-law formulation, it is
implicitly assumed that the rate of vaporization at the droplet surface is directly equal to the rate of
consumption of fuel at the flame sheet. Initially, little vapor exists in the gas phase and the flame is
positioned closer to the droplet surface to achieve stoichiometric consumption of fuel and oxidizer. The
close proximity of the flame to the droplet surface causes increased vaporization, resulting in an abundance
of fuel vapor between the droplet and the flame which causes the flame to, ultimately, move away from the
surface. In environments with low oxygen concentration, the flame position continuously increases while, in
environments with high oxygen concentration, the flame position reaches a quasi-steady value.
However, other limitations of classical theory can only be addressed by considering finite rate
chemical kinetics. In the 1970's Law used single-step activation energy asymptotic analysis to develop
ignition and extinction criteria. By considering single-step finite rate chemical kinetics with a high
activation energy, it is possible to obtain an entire family of steady state droplet combustion and vaporization
solutions dependent on a parameter known as the system Damköhler number, a non-dimensional ratio of the
characteristic flow time to the characteristic chemical reaction time of a given combustion system. In the case
of droplet combustion, the relevant Damköhler number is:
|
(5) |
where the characteristic flow time has been defined as the droplet radius squared divided by the characteristic gas phase mass diffusivity. Droplet combustoin parameters, for example burning rate, can be expressed as a function of this Damköhler number (Fig. 2.)
Figure 2 Characteristic S-curve of droplet characteristics for combustion and vaporization (Law, 1982).
As the Damköhler number approaches zero, solutions approach that of chemically
frozen vaporization, i.e. pure vaporization conditions. As the Damköhler number increases due to increased
droplet size or increased gas phase temperature, the burning rate slowly increases until a critical point is
reached. This corresponds to the critical ignition Damköhler number.
The upper right hand corner of the S-curve corresponds to infinitely fast chemistry and thus
corresponds to the d2-law solution. During quasi-steady combustion the droplet diameter decreases
resulting in a decrease in the system Damköhler number. As the characteristic gas-phase transport time
becomes comparable to the chemical reaction time an increase in the leakage of reactants through the reaction
zone causes a decrease in flame temperature and, ultimately, extinction of the envelope diffusion flame.
Flame extinction has been observed at finite droplet diameters by many investigators, but never with much
assurance the observations were not perturbed by other phenomena.
Typically, the problem is that the droplet diameter at extinction conditions is so small that it is
difficult to define the extinction condition, either by flame disappearance or by change in the rate of
vaporization. Both convection and residual enthalpy in the gas phase cause vaporization of very small droplets
to continue , often to completion
More recently, the effects of multi-step reduced chemistry and full detailed chemistry have been
examined, using rate ratio asymptotic theory. The claim of such theories is that more realistic finite
chemistry can be included. While such theories can qualitatively and even quantitatively reproduce some
of the experimental observations, for example burning rate or extinction diameter, with appropriate
selection of physical and chemical parameters, none have as yet quantitatively predicted all of these
experimental observables simultaneously with the same set of physical and chemical parameters.
Furthermore, the models are seldom predictive for variations in inert, for example, helium v. nitrogen,
without change in other parameters. None have yet included quantitatively the effects of radiative heat
loss shown to be important in the large-droplet experiments typically conducted in space experiments.
Difficulties will be experienced in including radiation as long as flame stand-off and temperature are
incorrectly predicted. Several researchers continue to apply complex mathematical theories such as rate
ratio asymptotics, to study various specific aspects of isolated droplet combustion, and to develop
empirical correlations for predicting isolated droplet combustion phenomena. The theories are extremely
useful in general qualitative terms and, under some circumstances for correlating experimental observations.
As with numerical models, the resulting empirical formulations for isolated droplet burning are not
directly applicable to the modeling of practical spray combustion phenomena.
Today, isolated spherically symmetric droplet combustion along, with one dimensional, laminar
premixed and diffusion flames, all represent fundamental combustion venues that can be time-dependently,
computationally modeled (even time dependently) with essentially no constraints on the functional property
dependence or level of descriptive detail for phase transformation, convection, diffusion, chemistry and
heat transfer. Robust sub-models for each of the physical or chemical processes involved can developed,
validated, and tested independently against other fundamental work (for example kinetics can be tested
against fundamental static reactor, flow reactor and shock tube experiments) and then their behavior and
interactions can be tested in concert with other sub-models, for example diffusive sub-models) by comparison
against experimental data for the above fundamental combustion venues. The interactions and the importance
of various sub-models and model components can be studied through formal mathematical as well as parametric
sensitivity studies, and empirical correlations can be derived to quantitatively predictive for the fundamental
combustion observations. Typically, the complexity of the sub-models are such that the same levels of
robustness are prohibitive computationally for multi-dimensional computational models for practical combustion
problems. Most importantly, simplification techniques for sub-models can also be developed and validated by
comparison with computations for the fundamental venues using the robust models. The simplified models can
then be utilized in complex multi-dimensional computational descriptions for practical combustion processes,
including spray combustion. It should be clearly understood that the experimental data and computational
components developed through research using fundamental venues is not directly transferable to practical
combustion analyses . For example, in the case of sprays, droplets seldom burn individually in such processes,
nor do they burn without interactions with other surrounding droplets. Some examples of numerical modeling
are presented under the current results section on this web page. More details can be found in the publications
listed on this web page.
Spherically Symmetric Experiments
The elegant classical and asymptotic solutions described above, as well as the detailed kinetic modeling discussed here are not possible currently without the major assumption of spherical symmetry of both the liquid droplet and the surrounding gas phase. Thus, of equal importance to the development of fundamental computational tools to model droplet combustion are fundamental spherically-symmetric, experiments which can be used to develop and validate the models. The combustion of a single isolated droplets, even in initially quiescent gases, typically generates sufficient heat to cause natural convective gas motions relative to the drop surface, thus destroying the spherical symmetry of the experiment. These motions result from the local gas temperature field and the gas motions induced by buoyancy effects. The importance of buoyancy can typically be inferred from the value from the magnitude of a non-dimensional grouping referred to as the Grashof number. The Grashof number can be thought of as a comparison between the characteristic buoyant velocity and the characteristic diffusion velocity:
|
(6) |
where 
is the characteristic density change,
is the mean density, g is the local acceleration due to gravity, L the characteristic
length scale (in this case the drop diameter), and Dg the mass diffusivity. Similarly, the
Richardson number can be thought of as a comparison between the characteristic buoyant velocity to
the characteristic convection velocity:
|
(7) |
For the effects of buoyancy to be small, conditions must be met such that both the
Grashof number and Richardson number are O(10-1).
In droplet combustion, (even in the spherically symmetric case) both molecular diffusion and bulk convection,
termed Stephan flow, (produced as a result of phase changes at the droplet surface) are important
phenomena. For vigorously vaporizing droplets, the Stephan flow velocity dominates over the diffusion velocity
near the droplet surface. Thus, if spherical symmetry is desired only in the vicinity of the droplet surface,
the relevant dimensionless parameter is the Richardson number. The Stephan flow at the droplet surface can be
determined from the conservation of mass at the surface to be:
|
(8) |
where rs is the droplet radius, the liquid density, and
the gas density at the vapor/liquid interface. For example, the combustion of a 1 mm
methanol droplet in air, yields a Stephan flow velocity at the droplet surface of about 0.16 m/s. At these
conditions, 
is approximately unity, such that the Richardson number is approximately
0.01, even at normal gravity. This suggests that near the droplet surface the gas phase is spherically
symmetric. However, the gas phase conservation of mass requires that 
= constant,
such that the Stephan flow velocity decays quickly at increasing gas-phase radii.
The characteristic diffusion velocity, meanwhile, does not decay with increasing radii and, thus, the
relevant parameter to determine spherical symmetry throughout the entire gas phase (for no forced convection)
is the Grashof number. For the same conditions as above, based on a (typical experimental) flame standoff of
about 5, the resulting Grashof number at normal gravity is approximately 1500. Thus, for a 1 mm droplet burning
in air at 1 atm, a gravity level of 10-4 is required to achieve a Grashof number of
O(10-1). It is interesting that droplets as large as 6 to 7 mm are of interest in experiments in
space, and these experiments are thus some of the most sensitive to background gravitational effects,
requiring gravity levels below 10-6 .
A variety of procedures and facilities exist to achieve reduced gravity levels. The earliest low gravity
experiments were first performed in drop towers by Kumagai in 1950. 1950. To date, microgravity experiments
have been conducted using drop towers, parabolic flight aircraft, sounding rockets, and orbiting spacecraft.
These experiments result in a variety of gravity levels and test times as summarized in Table 2.
Microgravity Facility Gravity Level Test Duration Drop tower 10-4 g - 10-6 g 1 - 10 seconds Parabolic flight aircraft 10-1 g - 10-3 g 5 - 15 seconds Sounding rockets 10-4 g 200 - 900 seconds Orbiting spacecraft 10-6 g 1000 - 10000 seconds
Based on the above Grashof number argument, for droplet sizes on the order of 1 millimeter and larger should only be accurate when conducted in drop towers or aboard orbiting spacecraft. The inherent complexity and uncertainty (a typical success rate of about 33% is standard for untethered experiments) of isolated droplet combustion experiments presents difficulties in performing these experiments in sounding rockets. In parabolic flight aircraft, the g-levels are too high to perform isolated droplet combustion experiments with accuracy; however, it is a very good means to test experimental operating systems and procedures.
It is also possible to reduce the effects of buoyancy by conducting experiments in normal gravity at reduced pressure and/or reduced initial droplet size. However, all droplet combustion experiments conducted under normal gravity are complicated by buoyancy and/or intrusion of a suspension device. Buoyancy enhances the gas phase heat and mass transport thereby increasing the burning rate. Since the flame shape is distorted, it is difficult, if not meaningless to define a flame position. Finally, there is an inherent effect of pressure on the chemical kinetics occurring in the flame structure. Since the effects of buoyancy vary with the droplet diameter, normal gravity experiments are completely transient. The presence of a suspension device, meanwhile, distorts the droplet shape and is an additional source of heat loss from the flame.